![]()
CHAPTER 5 - LANDSCAPE CHANGE DESCRIBED BY SELECTED METRICS
5.5. Landscape transformation in Machadinho and Anari
5.5.1. Metrics and meanings
This chapter presents results for landscape, class, and patch metrics, in a multi-temporal approach, for two distinct settlement designs in Rondônia, Brazilian Amazon. Perhaps, the most intuitive analysis of metrics occurs at the class level. Landscape metrics average values of all classes and can lead to misleading conclusions if not analyzed with caution. However, when comparing two landscapes of distinct structure, such as Machadinho and Anari, landscape metrics provide important elements for a description about the general pattern within the settlements. Patch metrics are too disaggregated and can be more useful when analyzing single patches for specific purposes (e.g., habitat studies, reserves delimitation, edge effects, and so on). The importance of patch metrics is mainly related to their role in providing the basis for class- and landscape-level calculations, as depicted by the formulas for these latter metrics (Appendix 2). However, the analysis of distribution of values for specific patch metrics can also contribute to a better understanding about the spatial pattern of landscapes, as patches are the primary elements defining landscape structure. When averaged to the class level, specific patterns within the landscape become clearer, particularly when analyzing LULC change as the most important process leading to landscape transformation.
Other techniques could be used to overcome the limits of this research. A promising study would be to measure the importance of specific groups of patches to processes causing changes in landscape pattern. In the case of roads and watercourses, for example, analysis of connectivity instead of patch distribution would be more appropriate since the design of these corridors ultimately affects landscape patterns and processes of change, as indicated by the analysis of buffers around roads in Chapter 4. Another empirical exercise would be to fill the reserves in Machadinho with a similar pattern found in property areas and simulate the inclusion of permanent reserves within the Anari landscape to test the actual contribution of reserves and architectural design to landscape fragmentation processes. Until these studies are carried out, the paragraphs below discuss the results produced so far, as a contribution to rethinking settlement design in the Amazon.
Results for Percentage of Land (PLAND) are redundant with the ones presented in Table 14, but in this chapter they are aggregated for the recoded classes (i.e., forest, succession, production, and others). Slight differences between these numbers and results presented in Chapter 4 are due to the use of an adjusted boundary for Anari when calculating landscape metrics.2 The classes used for this research have distinct functions within the landscapes. Further studies may be more specific about their role in flows of materials, energy, and species within landscape elements. PLAND is a very useful metric when comparing same classes between landscapes of different sizes, such as Machadinho and Anari. It is not inappropriate to reaffirm the importance of communal reserves in maintaining a higher percentage of forest cover in Machadinho. Without them, the rate of deforestation becomes similar in both landscapes (Table 28).
The Largest Patch Index (LPI) is one of the most effective metrics measuring landscape fragmentation (Dale 2001). At the landscape level, LPI decreases in all cases, but more abruptly in Machadinho without reserves and Anari (Table 39). This result is actually reflecting what happened to forest class, which shows an equivalent trend (Table 29). The relatively large size of the Aquariquara Reserve in Machadinho is affecting LPI results positively for this landscape. Certainly, the reserve itself and contiguous private forest areas make up the LPI value for forest in Machadinho. Interestingly, because of this communal reserve, this metric tends to remain stable in Machadinho, while it keeps decreasing in Anari.3 This has significant ecological implications, as some species need a single large patch as their primary habitat for maintenance and reproduction (Burkey 1989). LPI values for succession, production, and other features have relatively less importance because their largest patches are too small in comparison with the landscape's extent. However, the higher values of LPI for succession and production areas in Anari indicate that land aggregation (for pasture conversion, for example) and land speculation (through relative abandonment) is more current in the fishbone scheme.
Table 39 - Trends found for landscape metrics in Machadinho d’Oeste and Vale do Anari between 1988 and 1998.

Patch density, patch size, and patch variability metrics are other important quantitative measurements to assess landscape transformation and fragmentation because the total amount of energy and nutrients in a patch is proportional to its area (Forman and Godron 1986). The consequences of these matters to species composition and abundance within the landscape are clear. As pointed out by the island biogeographic theory, species diversity or richness is related directly to an island's area, its isolation, and its age (MacArthur and Wilson 1967). Patch size can stand for an island's area for analyses of species habitat (Harris 1984). It is out of the scope of this chapter to discuss the effects of patch size and variability on species. But it is important to mention that the study of landscape structure can effectively contribute to the understanding of occurrence and distribution of organisms (Ricklefs 1987).
Within the study area, results for Patch Density (PD) have to be considered with caution. Although higher in Machadinho at the landscape level (Table 27), this finding reflects the fragmentation of succession and production areas more than of forest stands. This is corroborated by the results of PD at the class level, which are more informative (Table 30). Production fields and succession areas in Machadinho are smaller and more numerous, increasing PD despite the lower level of fragmentation of forests within this landscape. When excluding reserves from the analysis, values of PD of forest in Machadinho are higher because the large patches of forest are not being considered in the calculation. Results also indicate trends in rates of forest fragmentation. PD of forest increased more than 2.5 times in Anari during the period of study, indicating a faster process of forest fragmentation (Table 40). PD is ultimately measuring landscape and class heterogeneity, providing important quantitative information for land zoning and management initiatives.
Table 40 - Trends found for class metrics in Machadinho d’Oeste and Vale do Anari between 1988 and 1998.
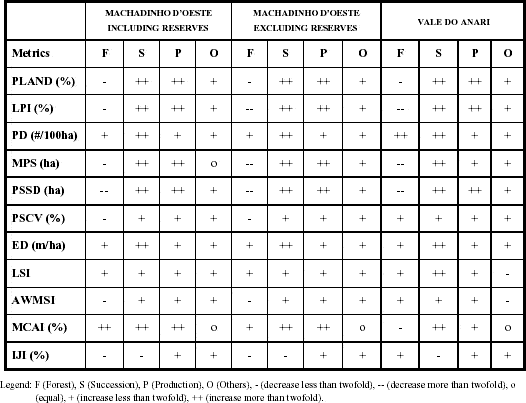
Mean Patch Size (MPS) is derived from patch-based area metrics and also indicates fragmentation. The first important finding within all cases under analysis is that MPS averaged for the entire landscape decreases over time (Table 39). Also, landscape MPS is lower in Machadinho, but decreases at a faster rate in Anari. To better understand these rates of fragmentation and which landscape elements are contributing to the process, analyses at the class level are more helpful. In general, MPS decreases for forest and increases for all other classes (Table 40). Moreover, a lower pace of forest fragmentation is indicated for Machadinho when compared to Anari. Although MPS of forest was smaller in Machadinho in 1988, it ended up larger after a decade of landscape transformation. This occurred because MPS of forest decreased 1.9 times in Machadinho and 4.4 times in Anari during the period of study! The fishbone scheme tends to show lower levels of fragmentation during the early stages of colonization due to the large elongated patches of forest located between roads. However, when forest clearing advances, these patches are subdivided into several smaller patches. In Machadinho, communal forest reserves combined with private property forests produced a more stable landscape. If the reserves are excluded, MPS of forest drops abruptly, suggesting a much more fragmented class than even the fishbone design. Obviously, the exclusion of reserves in Machadinho was just an empirical exercise. Nevertheless, the results offer an alert for further initiatives trying distinct settlement designs in the Amazon. MPS of successional vegetation and cropland are always greater in Anari through time. These trends are affected by the increase in pasture areas and associated secondary vegetation within the fishbone settlement. Results for MPS of succession, production, and other areas are unaffected when excluding reserves in Machadinho becau e these three classes are barely absent within the reserves. Conversely, results for patch-based and class-based area metrics for forest are always affected by the exclusion of reserves, indicating the importance of their patches for the statistical population and distribution of forest class within Machadinho's landscape (Figures 71, 72, and 73).
As an average, MPS is sensitive to extreme values. Thus, its results are better interpreted when analyzed together with measurements of dispersion. The behavior of Patch Size Standard Deviation (PSSD) and Patch Size Coefficient of Variation (PSCV) follows the trends observed for MPS. In general, these dispersion metrics decrease as MPS for forest decreases and increase as MPS for the other classes increases over time (Tables 39 and 40). In other terms, the variability in patch size of forest and landscapes as a whole is decreasing, making the distribution of these metrics more uniform. The only exception refers to PSCV of forest. As it is calculated as a percentage of MPS, it increases in Anari, reflecting a higher relative variability in size of forest patches within the fishbone landscape in 1998, even with a lower absolute variability measured by PSSD. On the other hand, PSSD and PSCV of production and succession classes increase over time, indicating that these areas are becoming larger, with different sizes (Tables 27, 32 and 33).
The next two groups of metrics (i.e., edge metrics and shape metrics) have important applications for the study of edge effects, which certainly affect the dispersal and foraging of organisms (Ranney et al. 1981, Laurance and Bierregaard 1997). Edge effects are important ecological phenomena and particularly useful for the study of rain forest fragments (Lovejoy et al. 1986, Malcolm 1994, Kapos et al. 1997, Laurance 1997). The amount of edge and the shape of patches dictate the interactions between distinct patch types and, consequently, the flow of species throughout the landscape. In this sense, a large but elongated patch, such as the strips of forest between roads in Anari, could become completely edge habitat. More than explore scenarios about edge effects occurring within the landscapes of Machadinho and Anari, this research provides a preliminary analysis of edges and shapes as a basis for further studies regarding ecotones and transitions among patch types. Comparative studies of species diversity and abundance in Machadinho and Anari, for instance, could offer new elements for discussion about settlement design.
The similarity found in Edge Density (ED) for classes in Machadinho and Anari suggests that fragmentation is taking place within both landscapes (Tables 34 and 40). In general, ED is increasing for all classes as the process of occupation and LULC change advances. The abrupt increase in ED when excluding reserves in Machadinho should not guide us to misleading conclusions. In this case, the results are affected by the reserves' edges, considered as background for calculations. Further studies about edges within those landscapes should explore the role of contrasts between different patch types. The absence of results for edge contrast metrics minimized the potential of indicators to analyze ecotones. Depending on the land-cover class adjacent to forest patches, for example, different effects may be observed in terms of ecological processes. If forest patches border open vegetation, such as production areas, this fragment may become more susceptible to disturbances in its structure and composition. Conversely, if forest patches are adjacent to succession areas, secondary regrowth may be accelerated. Investigating these relationships would bring a better understanding about the functional significance of each patch type. Such inferences go beyond the purposes of this study, but the exploratory results obtained for edge contrast metrics justify the use of these quantitative approaches in further ecological analyses within Amazonian landscapes under processes of LULC change.
Edge contrast and nearest-neighbor metrics were not calculated due to the computer intensity when processing large and complex data sets such as those used for this research. However, exploratory runs for different dates and subsets confirmed what was expected. Edge contrast metrics measure the degree of contrast between a patch and its neighbors by assigning different weights to classes within the landscape. Nearest-neighbor metrics reflect configuration by measuring the distance between nearest patches of the same class, based on edge-to-edge distance. In other terms, they indicate the degree of isolation of patches within the landscape. The following three metrics were used during this exploratory approach (McGarigal and Marks 1995). In general, Contrast Weighted Edge Density (CWED) tends to increase and Mean Edge Contrast Index (MECI) tends to decrease within Machadinho and Anari landscapes over time. Mean Nearest Neighbor (MNN) also tends to decrease, as these landscapes become more fragmented. The exploratory analysis of edge contrast and nearest-neighbor metrics at the class level indicated similar behavior for both landscapes, as found for landscape metrics. Although the magnitude of each metric for all dates was not computed, some general trends can be mentioned. CWED tends to increase for all classes while MECI tends to decrease, mainly for forest. Mean Nearest Neighbor (MNN) increases for forest and decreases for succession and production areas, as expected for an ecological process where a pristine landscape matrix is progressively subdivided into more isolated remnant fragments.
Shape metrics are also related to edge effects, as patch shape and size dictate perimeter extent and edge with neighbor patches. These perimeter-area relations are intricate to quantify concisely in a metric and often are difficult to interpret (McGarigal and Marks 1995). Patch perimeter distribution was represented in Figures 74, 75, and 76. Perhaps, the most important finding was that perimeter for forest patches in Anari shows a similar pattern in all dates, while in Machadinho it increases over time. The results obtained for the computed shape metrics show relevance related to some particular trends. Landscape Shape Index (LSI) increases over time for all classes and landscapes due to the formation of more irregular shapes (Tables 39 and 40). Values are higher for Machadinho because it has a more complex design. When excluding the reserves, these values are even higher for the same reason explained above for Edge Density (Tables 27 and 35). By the same token, lower LSI values in Anari indicate a lower complexity in patch shape within this landscape. When analyzing these results, it is important to consider that LSI is not measuring shape morphology. In this sense, a large elongated patch could have the same LSI value as a smaller convoluted patch. What LSI is in fact indicating is that the configuration of classes and landscape in Anari is less complex because its design is based on an orthogonal road network. In Machadinho, the design based on topography produces a more complex outcome in landscape shape structure.
When weighted by areas of patches, not just the magnitude but also the trends of shape metrics are different within the settlements. Area Weighted Mean Shape Index (AWMSI) for forest and the entire landscape decreases in Machadinho and increases in Anari during the period of study (Tables 27, 36, 39, and 40). The size and perimeter-to-area relationship of forest patches within the landscapes is certainly affecting the results, as AWMSI increases similarly for all other classes over time. Other quantitative analyses such as the interior-to-edge ratio relating edge and shape metrics could provide an easier intuitive interpretation. Although these relationships were not computed for this study, it is expected that communal reserves in Machadinho tend to increase the interior-to-edge ratio of forest while the narrow elongated forest remnants in Anari tend to lower the ratio for this patch type.
The analysis of shape metrics at the patch level is difficult to interpret, as even radical changes in the shape of some patches may have little effect on the distribution of patch shape values for the class as a whole. For metrics such as area and perimeter this is minimized because they are absolute values without limit and not ratios as the shape metrics are. However, results for the shape index of forest patches indicate a lower shape complexity of forest stands in Anari (Figures 77, 78, and 79). In addition, results for fractal dimension of patches of forest, succession, and production areas behaved as expected and described in previous studies (Frohn 1998). Forest patches tend to have more complex shape than agricultural or successional fields (Figures 80, 81, and 82).
Core-area metrics are the counterpart of edge metrics. They are related to the concept of 'interior habitat,' which is very relevant for a number of species (Patton 1975, Saunders et al. 1991). My decision to choose Total Core Area Index (TCAI) and Mean Core Area Index (MCAI) was to avoid redundancy with patch size, density, and variability metrics, as core area is generally a function of these latter measurements. TCAI and MCAI are relative indices that quantify core area as a percentage of total area (Appendix 2). All these metrics are based on the selection of an edge width, which should be associated with the phenomenon under investigation. As this research is related to processes of LULC change, the choice of an edge width of 90 m was based on potential responses of plants and the environment when subjected to LULC edge effects (Kapos et al. 1997). This decision is somewhat arbitrary, and empirical tests could clarify the effects of changing edge width to core-area metrics values. However, for the comparative purpose of this study, the results are already valuable.
Within the study area, TCAI decreases for all cases (Table 39), suggesting that the landscapes are losing interior habitat as they become more fragmented. Although TCAI is greater in Anari in 1988 because of the large elongated patches of forest, it decreases more slowly in Machadinho including reserves (Table 27). Also, lower values of TCAI in Machadinho when excluding reserves indicate that they play an important role in maintaining interior habitat within the landscape.
MCAI results should be analyzed with caution, as any metric based on first-order statistics. Interesting results were found for MCAI of forest. In Anari, the average of core areas represent 12.5% of the forest class in 1988, but drops to 6.4% in 1998. In Machadinho, MCAI of forest represents just 4.1% of this class in 1988, but increases more than twofold to 8.8% in 1998 (Tables 37 and 40). The meaning of these results can be better interpreted in conjunction with the percentage of the landscape covered by forest (Table 28). Although deforestation is a concurrent process within both landscapes, the architectural design of Machadinho preserves more interior habitat, which relatively increases as the area of forests decrease. This is independent of the large patches of communal reserves and strictly related to the intricate design of Machadinho. Conversely, the initially lower fragmented patches of forest in Anari have a relatively higher percentage of interior habitats in earlier stages of colonization, which drops abruptly as the occupation process takes place.
Diversity metrics brought little new information about landscape pattern and process in Machadinho and Anari. Often criticized for not providing information on the actual composition of a landscape and its elements, these metrics were used solely as a summary about richness and evenness within the study area. Therefore, MSIDI results imply that Machadinho is a less diverse landscape or, in other words, has fewer classes per unit area than Anari. When excluding the reserves, the results for MSIDI in Machadinho are similar to those in Anari, indicating that the diversity within private properties is equivalent in both landscapes. Results for MSIEI suggest that the proportional distribution of area among classes in Machadinho is less equitable than in Anari or in Machadinho excluding reserves (Table 27). Also, MSIEI could be understood as the compliment of dominance (that is, evenness = 1 - dominance). Although diversity metrics do not convey any information about the contribution of each patch type to the final result, analysis of these metrics in conjunction with other metrics (e.g., PLAND) may provide a better perspective about the trends being analyzed. In this sense, lower values of MSIEI in Machadinho reflect a higher dominance of forest within this landscape. By the same token, the ascendant trend for MSIEI in all cases (Table 39) depict the process of deforestation toward landscapes with a more even distribution of patches among forest, succession, and production areas.
The last metric computed for this comparative study was the Interspersion and Juxtaposition Index (IJI). The classic and intuitive way of representing maximum interspersion is by a chessboard, where white cells are evenly distributed in relation to black cells. IJI results indicate that Machadinho's design leads to a more interspersed landscape than Anari's fishbone scheme. They also indicate that the highest interspersion during the period of analysis occurred in 1994 (Table 27). IJI results for forest and production areas follow a similar trend, with lower values in 1988 and 1998, and higher values in 1994. This possibly indicates a threshold in the trajectory of colonization and landscape transformation within the study area. On the other hand, values for succession areas show a decreasing trend in all cases, suggesting that these areas are becoming more isolated within the landscape (Tables 38 and 40). The impact of these spatial relationships to ecological processes is a promising subject for further studies. Particularly, it is important to follow the trends for forest and succession areas and understand the potential impact of patch location within the landscapes to processes such as vegetation recovery or degradation. For these studies, it will be relevant to consider that forest fragmentation in both settlements may affect the propagation of disturbances across the landscapes. For instance, a highly fragmented and interspersed forest, taken as a patch type within the landscape, may be less prone to total destruction by fire as a class in its entirety, although the fragments themselves are more susceptible. For other types of disturbances (logging, for example), larger patches of forest may show a higher resilience than several small fragments. These processes should be investigated and monitored for each landscape through time since they are affected by settlement design, as translated by the metrics discussed in this chapter.
5.5.2. Unresolved problems in spatial data analysis
The search for quantitative methods to analyze and describe the structure of landscapes has become a high priority in landscape ecology (Turner and Gardner 1991, Wiens and Moss 1999). In addition, within a science still dominated by empirical approaches and case studies, the need of standardization is urgent. At least four potential methodological pitfalls should be addressed when analyzing spatial data: the boundary problem, the scale problem, the problem of modifiable units, and the problem of pattern (Burt and Barber 1996).
The boundary problem is related to the extent and location of the boundary of a study area, as well as the placement of the internal boundaries in an areal design (Wiens et al. 1985). The choice of boundaries is particularly important in landscape ecology, because the behavior of landscape metrics is affected by changes in spatial extent (Turner et al. Effects 1989). This was one of the first questions when designing the comparative analysis about landscape fragmentation processes in two distinct rural settlement designs in Rondônia. Which limits should be chosen? Where should the subsets be placed? Should the subsets have the same shape and extent? What will be the effects of boundary placement over landscape, class, and patch metrics?
One option was to choose subsets of exactly the same shape and extent. However, the settlements have completely different designs (Figure 6). Any shape would embrace more than the total area of the settlements or just a part of them. The landscape metrics calculated for these subsets would be strongly affected by segregation or integration problems, not allowing reasonable comparisons between the settlements as a whole. The other option was to digitize the geographic limits of each settlement and calculate the metrics in relation to their extent. Although the shape and extent of the subsets are different, comparative analyses become possible. The boundary problem in this case seems to be avoided, as the unit of analysis is the entire extent of the settlement, which is functionally circumscribed by administrative limits. Besides taking this option for the study, metrics with absolute values, such as number of patches or total area, were avoided to maintain consistency during the comparative approach.
The second problem when analyzing spatial data is related to the grain and is also called the scale problem or the areal aggregation problem (Cao and Lam 1997). In general, spatial aggregation tends to reduce the variation in spatial mosaics (Burt and Barber 1996). This is perhaps one of the most important issues in global change-related research, as scaling up and down is generally suggested (Curran 1989, National Research Council 1998). The qualitative and quantitative changes in measurements across spatial scales differ depending on how scale is defined. Therefore, measurements carried out at different scales may not be comparable. Also, the exact relationship varies across landscapes, creating difficulties in extrapolating from one region to another (Meentemeyer and Box 1987, Wiens 1989). Diversity metrics, for example, decrease linearly with increasing grain size, while dominance and contagion do not show a linear relationship. Rare classes are lost as grain becomes coarser and dispersed classes are lost more rapidly than clumped ones (Turner et al. Effects 1989).
Recognizing that landscape structure varies with scale, landscape ecologists have struggled for scale-invariant measures or indices (Withers and Meentemeyer 1999). The fractal dimension is the most commonly employed such measure. The range of spatial extent over which the fractal dimension is a constant is said to represent the 'scale' of the landscape, or the scale over which the landscape is 'self-similar' (Burrough 1981, Mandelbrot 1983). In other words, over that range of scales, landscape units display similar behavior, appear structurally similar, and are, presumably, affected by the same processes and controls. Other approaches, such as the square-pixel metric, have also been suggested for this purpose when dealing with raster data (Frohn 1998). An alternative measure for shape complexity is the lacunarity index, which is a multi-scaled method for determining the texture associated with patterns of spatial dispersion (e.g., landscape/habitat types or species locations) (Plotnick et al. 1993). Another approach is to identify the scale of discontinuity in landscape structure, or assess the variability or similarity between landscape types or patches. These measures include spatial autocorrelation (Legendre 1993), semivariograms (Curran 1988), and other geostatistical methods (Isaaks and Srivastava 1989, Rossi et al. 1992). A variety of complementary methods have also been developed. Fractal models of landscape patterns have been associated with neutral models of species co-occurrence (Milne 1992). Nested sampling designs have enabled the detection of a wide range of spatial structures, showing the relationships among nested spatial scales (Bellehumeur and Legendre 1998). Hierarchically structured maps have been suggested as a useful tool for studying landscape patterns at different scales (Lavorel et al. 1993). In sum, the need for a 'scale theory' has been defended frequently as an important methodological advance in spatial analysis (Raffy 1994).
For this research in Rondônia the scale problem was minimized using the same grain for both areas of study. The areas are adjacent and belong to the same classified Landsat TM scene. Therefore, comparisons between the areas were possible, as the agroecological processes under investigation (e.g., deforestation, secondary succession, and land conversion to pasture or agriculture) were studied using the same spatial resolution. Potential problems may arise if integration of spatial data produced at other scales is done (e.g., soil maps, topographic features, and so on). In this case, caution should be taken to keep away from biased estimations.
A third potential problem when dealing with spatial data is associated with modifiable units: results vary when areal units are progressively aggregated into fewer and larger units of analysis (Turner et al. Effects 1989). This may happen even when using the same grain and extent for the analysis. Burt and Barber (1996) explain how variance may or may not vary depending on the aggregation process. In general, smoothing techniques decrease variance and also increase autocorrelation (Bian 1997). However, the effects of using modified areal units are not always predictable. A rule of thumb to minimize the problem when aggregating data is to join zones with similar attributes (Bian and Butler 1999). This problem is typically recognized when classifying categorical data using hierarchical schemes such as Anderson's LULC system (Anderson et al.1976). Joining distinct classes must be done carefully to avoid an undesirable significant decrease in spatial variance. Important components to consider include the nature of the classification scheme itself, the process of classification output issues, and the phenomenon under investigation (Withers and Meentemeyer 1999).
For this research in Rondônia, image classification was done using the same parameters and spatial-spectral relations for both study areas. Just after obtaining the LULC map for the entire scene, the settlements were separated to run the landscape metrics. This method avoided incomparable approaches between the sites. Difficulties to discriminate some LULC types were solved by analysis of vegetation structure and spectral data, as discussed in Chapter 3. Aggregation of classes for the LULC analysis in Chapter 4 or for the classes used in this chapter was strictly based on functional aspects of each category within the classification system. Although the accuracy achieved using maximum likelihood algorithms was acceptable (Table 13), further approaches may improve the accuracy of LULC classifications for the study area. Spatial autocorrelation studies may support decisions about the classification system and the relationship of neighboring attributes within data elements (Legendre 1993). The use of semivariograms may help the detection of areas with higher chance of showing the modifiable areal unit problem (Curran 1988). Spatial-spectral algorithms may overcome the risk of joining very dissimilar categories (Kettig and Landgrebe 1976, Landgrebe 1980, Woodcock and Strahler 1987).
The pattern in spatial data is another problem for several methods of analysis. Many of them are incapable of assessing the type of pattern present in a spatial distribution (Burt and Barber 1996). Landscape ecologists have also attempted to address this fact when using landscape metrics. In this case, an extra effort has to be made to depict landscape configuration besides describing landscape composition (Li and Reynolds 1993). One way to address the problem is to use second-order statistics methods (e.g., Ripley's K, Moran's I, Geary's c, semivariance, among others). They allow the quantification of small-scale spatial pattern intensity (magnitude, degree) and scale (spatial extent). But, again, these methods were primarily implemented for point data, under the assumption of stationarity. A shortcut to analyze patch data using spatial statistics algorithms is through surface pattern methods, such as join-count spatial correlation coefficients, in which patch centroids can be analyzed with point-pattern methods (Fortin 1999). Perhaps, the landscape metrics more suitable to address pattern in patchy spatial data are contagion and interspersion indices. Contagion measures both patch type interspersion (i.e., the intermixing of units of different patch types) as well as patch dispersion (i.e., the spatial distribution of a patch type) (Li and Reynolds 1993). The interspersion index measures the extent to which patch types are interspersed (i.e., adjacent to each other). The interspersion index is not directly affected by the number, size, contiguity, or dispersion of patches per se, as the contagion index is (McGarigal and Marks 1995). Alternative metrics to quantify contagion have also been suggested (Frohn 1998).
For the research in Rondônia, besides the analysis of landscape composition within the two settlement designs, it was important to investigate the variation of spatial arrangement through space and time. Distinct land-use strategies and consequent land-cover spatial outcomes were depicted from this analysis. However, one of the main shortcomings of interspersion metrics, for example, is their capability of analyzing spatial pattern based only on relationships between neighboring zones. More complex spatial relationships involving distant patches are still not implemented.
Last but not least, studies have shown high correlation among particular landscape metrics (e.g., Hargis et al. 1998). Caution should be taken to look for complementary techniques when analyzing spatial data, avoiding redundancy when it is not required. Primarily, besides the constraints of operational limitations, the choice of metrics should be strictly related to the phenomena under investigation. This chapter intended to provide elements for discussion regarding landscape change in the Amazon through the use of quantitative methods of spatial analysis. The next chapter goes beyond the metrics to address the human dimensions of landscape change.